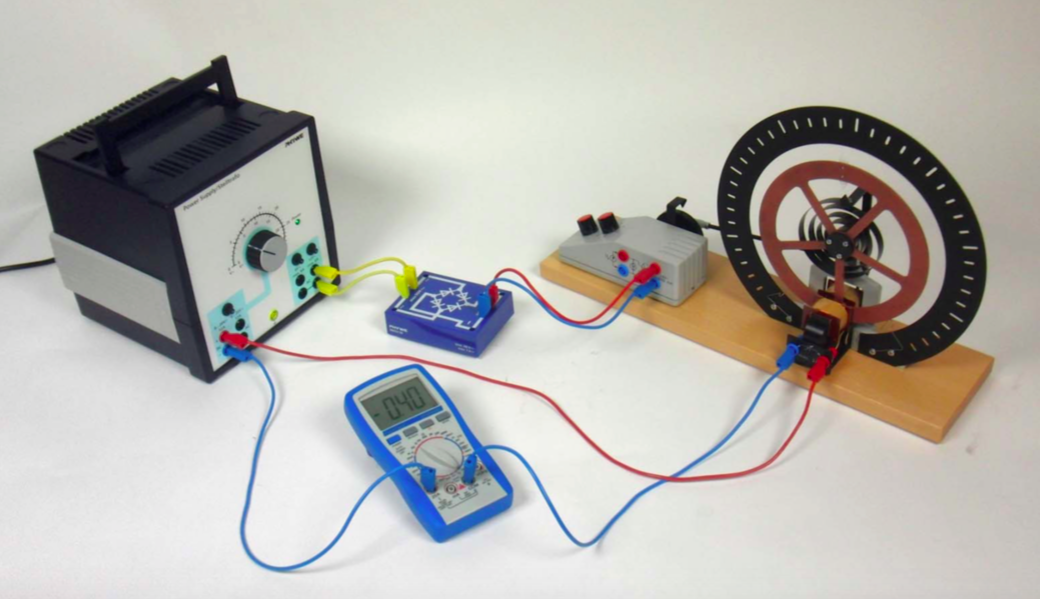
Item no.: P2132701
Principle
If an oscillating system is allowed to swing freely it is observed that the decrease of successive maximum amplitudes is highly dependent on the damping. If the oscillating system is stimulated to swing by an external periodic torque, we observe that in the steady state the amplitude is a function of the frequency and the amplitude of the external periodic torque and of the damping. The characteristic frequencies of the free oscillation as well as the resonance curves of the forced oscillation for different damping values are to be determined
Tasks
- A. Free oscillation
- To determine the oscillating period and the characteristic frequency of the undamped case.
- To determine the oscillating periods and the corresponding characteristic frequencies for different damping values. Successive, unidirectional maximum amplitudes are to be plotted as a function of time. The corresponding ratios of attenuation, the damping constants and the logarithmic decrements are to be calculated.
- To realise the aperiodic case and the creeping.
- B. Forced oscillation
- The resonance curves are to be determined and to be represented graphically using the damping values of A.
- The resonance frequencies are to be determined and are to be compared with the resonance frequency values found before hand.
- The phase shifting between the torsion pendulum and the stimulating external torque is to be observed for a small damping value assuming that in one case the stimulating frequency is far below the resonance frequency and in the other case it is far above it.
What you can learn about
- Angular frequency
- Characteristic frequency
- Resonance frequency
- Torsion pendulum
- Torsional vibration
- Torque and restoring torque
- Damped/ undamped free oscillation
- Forced oscillation
- Ratio of attenuation/ decrement
- Damping constant
- Logarithmic decrement
- Aperiodic case
- Creeping